
Formulae, unlike text, offer no interpretive leeway
Review of the exhibition Bernar Venet. Painting: From Rational to Virtual. 1966–2024. at the Art Museum Riga Bourse
In a private conversation at the French Embassy in Riga, conceptual artist Bernar Venet (b. 1942) was asked whether his use of mathematical formulae in his paintings had personal significance. His reply was disarmingly candid: as a young man, he had hated mathematics.[1]
This anecdote captures the paradoxical relationship between Venet’s personal history and his artistic practice. According to American art critic Thomas McEvilley, Venet grew up in Saint-Auban, a small industrial town in the Alps of Haute-Provence. Raised in a working-class family, Venet’s artistic journey began in earnest when, at 17, he met the artist Arman. This pivotal connection introduced him to the École de Nice, a loosely defined movement that included figures such as Yves Klein and Arman. While the group aligned itself with Nouveau Réalisme, it was explicitly anti-Parisian, rejecting what its members viewed as the stagnation of modernist orthodoxy in the capital.[2]

Venet’s divergence from Yves Klein’s metaphysical monochromes is especially notable. Rejecting notions of transcendence, Venet adopted a constructivist sensibility. Viewing Venet’s oeuvre within a broader international context of the period, it is evident that his paintings have engaged with—while also resisting—movements such as Minimal Art, Color Field painting, and Pop Art, as well as the dematerialization discourse of Conceptual Art.

In his latest exhibition, Painting: From Rational to Virtual, 1966–2024, hosted by the Riga Bourse, Venet presents a compelling exploration of the ambiguous aesthetics of his work. The term “ambiguous” here underscores the duality of his paintings: their surfaces may evoke the sleek decorativeness of a polished coffee table or the detached formalism of a mathematical diagram transcribed onto canvas. Yet beneath this apparent simplicity lies a profound and interdisciplinary engagement with semiotics and linguistics.

Take, for instance, the work titled Gold Saturation with 45. This large-scale piece features a circular canvas coated in gold acrylic, framed in violet. Associatively, the round form suggests the mundane functionality of an outdoor table or perhaps a shield with a central hole—a visual trope verging on kitsch. Yet, the composition resists this reductive reading through its deliberate interplay of text in varying fonts, colors, and sizes. The result is a collision of high and low cultural registers. The mode of painterly execution is reminiscent of the “neutralized” brushstrokes of Jasper Johns. Furthermore, the surface is coated with varnish, which renders it even less expressive.

Venet’s characteristic use of mathematical equations, engineering schematics, and graphs as both form and content further destabilizes conventional understandings of artistic representation. Unlike Pop Art’s ironic appropriation of cultural symbols, Venet employs mathematical formulae as a deliberate strategy to eschew subjectivity. Formulae, unlike text, offer no interpretive leeway; their meaning is fixed and universal. By deploying them as a visual language, Venet innovatively reframes the dichotomy between text and image.

Consider Parabole de la function y=2x²+3x-2 (1966), one of Venet’s earlier works. The painting features a monochromatic white field bisected by a black diagonal, functioning as a baseline for the sliding mathematical equations. The whites are executed with horizontal brushstrokes, again in a kind of degree-zero mode, whereas the black diagonal is applied mechanically, perhaps with the help of a ruler. The stark composition evokes a minimalist winter landscape, where the black silhouette contrasts sharply with the white background. The work’s degree-zero aesthetic reinforces its conceptual rigor, yet the overall effect is surprisingly immersive. The viewer is invited to “drown” in the visual and intellectual density of the piece, experiencing mathematics not as abstraction but as embodied form.

Venet’s modernist impulse—his adherence to the notions of self-referentiality and the rejection of metaphor—aligns with Greenbergian traditions. However, his concept of self-referentiality is narrower and more technical, aligning with the language philosophy of figures like Nelson Goodman. Goodman’s Languages of Art: An Approach to a Theory of Symbols (1968) provides a useful framework for analyzing Venet’s work, particularly in its exploration of the differences between textual and visual symbols. Goodman characterizes images as “dense” and non-notational, lacking the clear syntax and differentiation of text.[3] Venet’s paintings, however, destabilize this binary: his formulae are inherently textual, adhering to strict syntactic rules, yet their translation into visual form imbues them with ambiguity and density. Changes in scale, placement, or texture transform their meaning, compelling the viewer to engage not just with their scientific precision but also with their aesthetic materiality.
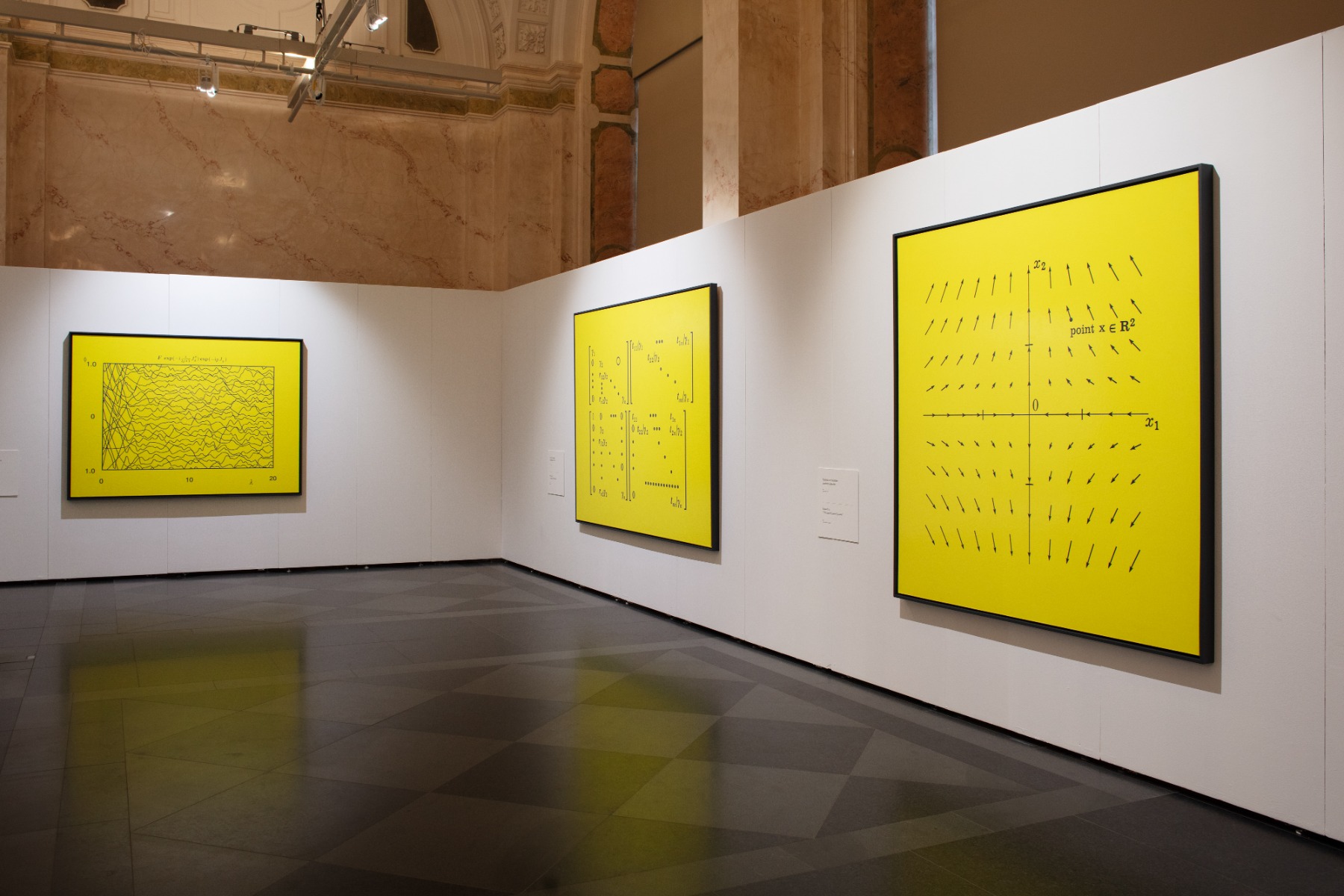
This hybrid symbolic system—part textual, part visual—positions Venet’s work within a broader knowledge acquisition discourse. His paintings suggest a convergence of science, industry, and art into a dynamic network of meaning. Bruno Latour’s actor-network theory, which emphasizes the interplay of human and non-human agents in constructing meaning, provides a compelling lens through which to view Venet’s practice. His use of mathematical principles does not merely illustrate scientific concepts; it transforms them into embodied, sensory experiences. Venet’s art thus proposes a new modality of knowledge—one that bridges the intellectual and the affective, the precise and the ambiguous.
***
[1] Bernar Venet, private conversation with the author, 24 January 2025.
[2] Thomas McEvilley, Bernar Venet: The Paradox of Coherence (Institute, 2010), 173.
[3] Nelson Goodman. Languages of Art: An Approach to Theory of Symbols (Bobbs-Merrill, 1968), 226–227.